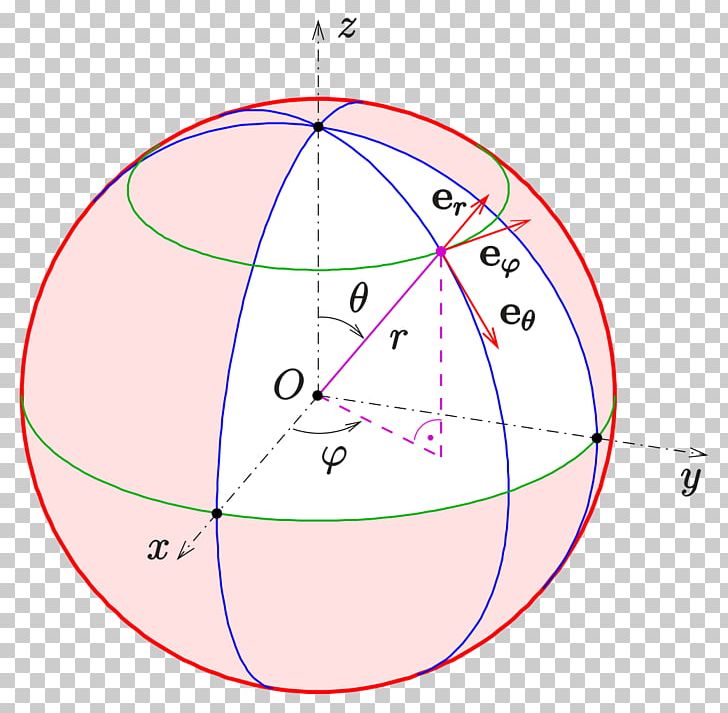
klionpatent.blogg.se - Sphere geometry calculator

Sphere geometry calculator how to#

Volume_sphere(`3`), returns `36pi` Calculate online with volume_sphere (volume of a sphere) To calculate, for example, the volume of a sphere of radius 1 + x, you must enter the following formulaĪfter calculating the result is returned. The volume calculator is able to do symbolic calculations in other words to do literal calculations. Symbolic computing the volume of a sphere Thus, calculating the volume of a sphere of radius 3 is done by typing the following formula The exact result and the approximate results are returned. The volume calculator is able to calculate the volume of a sphere, from a numeric radius, The volume calculator supports numeric but also literal expressions. The volume of a sphere is given by the formula `4/3*pi*r^3` where r is the radius of the circle. The calculator is able to calculate online the volume of a sphere, in other words, to calculate the The base is in the shape of a square, so A(base) = l².The online calculator allows to calculate the volume of a sphere from its radius. A = l × √(l² + 4 × h²) + l² where l is a base side, and h is a height of a pyramidĪ = A(base) + A(lateral) = A(base) + 4 × A(lateral face).The formula for the surface area of a pyramid is: That's the option that we used as a pyramid in this surface area calculator. Regular means that it has a regular polygon base and is a right pyramid (apex directly above the centroid of its base), and square – means that it has this shape as a base. But depending on the shape of the base, it could also be a hexagonal pyramid or a rectangular pyramid one. When you hear a pyramid, it's usually assumed to be a regular square pyramid. A = π × r × √(r² + h²) + π × r² given r and h.Ī pyramid is a 3D solid with a polygonal base and triangular lateral faces.A = A(lateral) + A(base) = π × r × s + π × r² given r and s or.Finally, add the areas of the base and the lateral part to find the final formula for the surface area of a cone:.Thus, the lateral surface area formula looks as follows: R² + h²= s² so taking the square root we got s = √(r² + h²) But that's not a problem at all! We can easily transform the formula using Pythagorean theorem:

Usually, we don't have the s value given but h, which is the cone's height.

(sector area) = (π × s²) × (2 × π × r) / (2 × π × s)įor finding the missing term of this ratio, you can try out our ratio calculator, too! (sector area) / (large circle area) = (arc length) / (large circle circumference) so: The formula can be obtained from proportions, as the ratio of the areas of the shapes is the same as the ratio of the arc length to the circumference: The area of a sector - which is our lateral surface of a cone - is given by the formula:Ī(lateral) = (s × (arc length)) / 2 = (s × 2 × π × r) / 2 = π × r × s The arc length of the sector is equal to 2 × π × r. It's a circular sector, which is the part of a circle with radius s ( s is the cone's slant height).įor the circle with radius s, the circumference is equal to 2 × π × s. Let's have a look at this step-by-step derivation: The base is again the area of a circle A(base) = π × r², but the lateral surface area origins maybe not so obvious:

A = A(lateral) + A(base), as we have only one base, in contrast to a cylinder.

We may split the surface area of a cone into two parts:

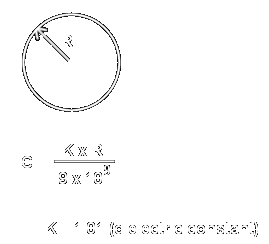
Sphere geometry calculator how to#

Surface area of a pyramid: A = l × √(l² + 4 × h²) + l², where l is a side length of the square base and h is a height of a pyramid.īut where do those formulas come from? How to find the surface area of the basic 3D shapes? Keep reading, and you'll find out! Surface area of a triangular prism: A = 0.5 × √((a + b + c) × (-a + b + c) × (a - b + c) × (a + b - c)) + h × (a + b + c), where a, b and c are the lengths of three sides of the triangular prism base and h is a height (length) of the prism. Surface area of a rectangular prism (box): A = 2(ab + bc + ac), where a, b and c are the lengths of three sides of the cuboid. Surface area of a cone: A = πr² + πr√(r² + h²), where r is the radius and h is the height of the cone. Surface area of a cylinder: A = 2πr² + 2πrh, where r is the radius and h is the height of the cylinder. Surface area of a cube: A = 6a², where a is the side length. Surface area of a sphere: A = 4πr², where r stands for the radius of the sphere. The formula depends on the type of solid. Our surface area calculator can find the surface area of seven different solids.